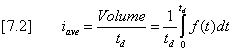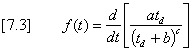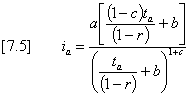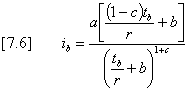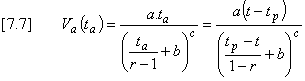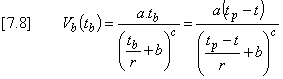|
|
|
Hydrological Theory
The synthetic hyetograph computed by the Chicago method is based on the parameters of an assumed Intensity-Duration-Frequency relationship, i.e.
where
i
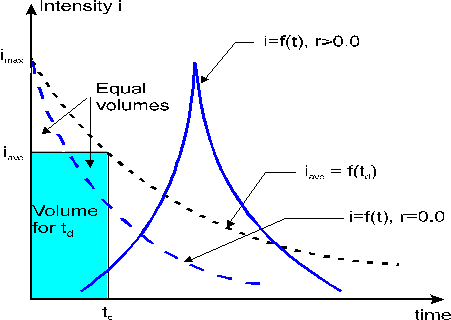
= average rainfall intensity (mm/hr or inch/hr) The asymmetry of the hyetograph is described by a parameter r (where 0 < r < 1) which defines that point within the storm duration td at which the rainfall intensity is a maximum. Imagine a rainfall distribution (with respect to time) such as that shown by the dashed curve of Figure 7-1, i.e. with a maximum intensity imax at the start of rainfall at t=0, which then decreases monotonically with elapsed time t, according to some function f(t) which is, as yet, unknown. If the duration of such a storm is td then it is easy to see that the total volume of rainfall is represented by the area under the curve from t=0 to t=td. The average rainfall intensity for such an event could be estimated as iave = Volume/td as illustrated by the shaded rectangle of Figure 7-1.
Figure 7-1 - Development of the Chicago storm Several storms with different durations td but with the same time distribution of intensity would produce values of iave which decrease as td increases, leading to the dotted curve of Figure 7-1. Thus:‑
If the average intensity iave over an elapsed time t can be described by an empirical function such as equation [7-1], then by combining [7-1] and [7-2], the functional form of f(t) can be obtained by differentiation, i.e.
or
Now if the value of r is in the range 0<r<1 the time to peak intensity for a given duration is tp = r.t The time distribution of rainfall intensity can then be defined in terms of time after the peak ta = (1-r).t and time before the peak tb = r.t by the following two equations.
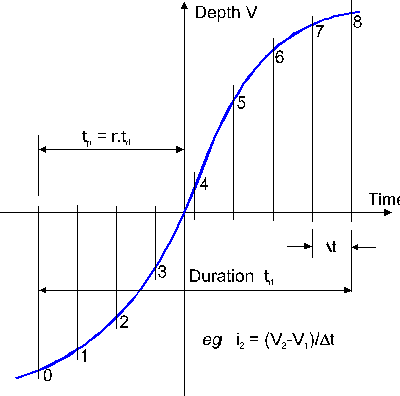
The solid curve of Figure 7-1 shows the time distribution of rainfall using a value of r greater than zero (r = 0.4 approximately). Calculation of the discretized rainfall hyetograph is carried out by integrating these equations to obtain a curve of accumulated volume as illustrated in Figure 7-2 below. For convenience this curve is computed so that volume V is zero at t=tp and is defined in terms of the elapsed time after and before tp. The expressions for volume after and before tp are then given by equations [7-7] and [7-8] respectively.
Figure 7-2 – Discretization of an integrated volume curve. Discretized values of rainfall intensity can now be obtained by defining a series of 'slices' of equal timestep Dt. The time step at the peak intensity is positioned relative to the tp position so that it is disposed about the peak in the ratio r to (1-r). In general, this means that the commencement of the storm may not be precisely defined by t = ‑ r td and the storm duration is therefore not disposed about the peak exactly in the ratio r to (1‑ r). However because the rainfall intensities at the extremities of the storm are generally very small this approximation is unlikely to lead to significant error.
|
|||
|
|
|||
|
(c) Copyright 1984-2023 Alan A. Smith Inc. |
|
|